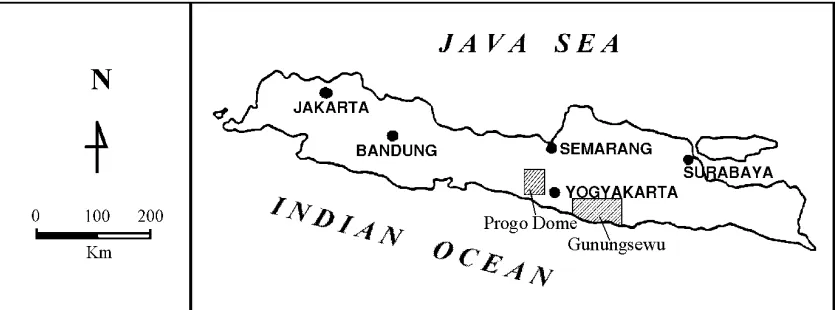
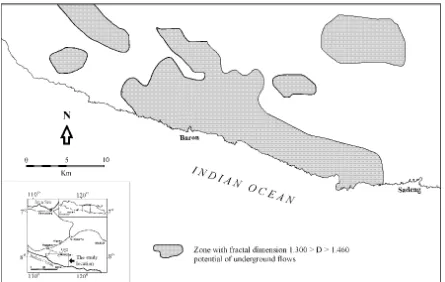
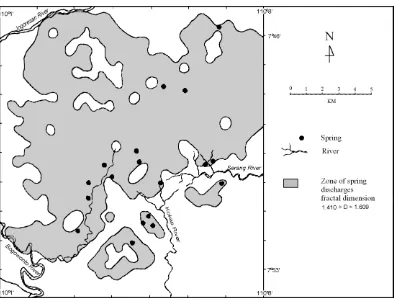
The application of fractal geometry analysis to groundwater exploration - Eprints UPN "Veteran" Yogyakarta
Teks penuh
Gambar

Dokumen terkait
Perbandingan dilakukan dengan perhitungan biaya dengan menggunakan asumsi yang dibandingkan dengan total tagihan listrik pihak manajemen Quest Hotel Surabaya dalam
Interaksi yang terjadi pada perusahaan berkaitan dengan struktur organisasi adalah sebagai berikut.. Hubungan Struktur
[r]
kiri berada di depan dada dengan punggung tangan menghadap ke luar, sedangkan.. tangan kanannya lebih tinggi ke atas menunjuk dengan semua jari ke arah lain.. dengan punggung
The Role Of Video Animation On students Concept Mastery In Title Separation Of Pure substances and Mixtures. Universitas Pendidikan Indonesia | repository.upi.edu
[r]
3.3.2 Prosedur penyusunan Neraca dalam Laporan Keuangan Pada Dinas pariwisata dan Kebudayaan Provinsi Jawa Barat Tahun 2010. Berikut ini adalah prosedur penyusunan Neraca
Sesuai dengan hasil evaluasi kelompok kerja, maka perusahaan Saudara merupakan salah satu penyedia jasa untuk diusulkan sebagai calon pemenang pada paket tersebut di atas, bersama